Pythagoras
 The Master Kuthumi as Pythagoras, Greek Philosopher and
Mathematician (582?-500?BC)
The Master Kuthumi as Pythagoras, Greek Philosopher and
Mathematician (582?-500?BC)
This page holds several stories about Phythagoras, each one a bit different. So please excuse us if the information is repeated.
Pythagoras was born in Samos on November 26th, 582 B.C. He entered the Order at Thebes on the second of April, 531, and having passed through all the initiations and examinations he entered the Illuminati, October 16, 529, and left at once for Crotona (Krotono), Italy, with jewels and documents to found a Grand Lodge there. There were a few so-called secret cults in existence at that time in Italy, and when Pythagoras began to promulgate his plans and admitted that women might not only be- come members, but could hold office, he attracted the attention of the most advanced thinkers of the day. Theano, the wife of Pythagoras, was one of the principal officers for three years. The Grand Lodge eventually had 300 brothers and sisters and issued many charters for local lodges of the order throughout Italy.
From this time onward toward the Christian period, great minds from many countries journeyed Eastward and Westward and Crossed the Threshold, and having completed the work and studies, passed again into the world's darkness to spread the light as they interpreted it.
As a historical record and a guide to the student who delights in research and antiquarianism, there will be given the names of those who came to Thebes to study, became Masters of Rosicrucian Lodges in other lands, and during their lifetimes published at least one book, an oficial work, treating on the Rosicrucian philosophies or sciences.
Many of the books or manuscripts to be listed are still extant in the original, or translated, and quite a few of them are in America. A perusal of any one of them convinces one of the author's real knowledge and experience in Rosicrucianism.
{p. 75} OUTER activities of the Great White Brotherhood, during the pre-Christian Era, were centered in a number of branches controlled by one group of supreme officers who constituted the Rosicrucian Fraternity of Brethren of the Rosy Cross. The Supreme Masters of the Great White Brotherhood withdrew from public activity and with a council of eminent advisors constituted the esoteric body known thereafter as the Great White Lodge.
The first spread of Rosicrucianism to the Western world was from the great seats of learning of ancient Egypt, namely, Tell el-Amarna, Thebes, Heliopolis, and Alexandria. The great masters, sages, or Kheri Hebs (high priests), who presided over the instruction, were initiates of the Great White Brotherhood. They authorized eminent scholars as disciples to go forth and disseminate the light under various organization names. Even those in authority, who were not initiates, acknowledged the greatness of the Secret Wisdom in the archives of the order, and appealed for its release to the worthy, as we shall see.
Philadelpllus, the Ptolemy principally responsible for the establishment of the first great university at Alexandria, Egypt, about 305 B.C., sought in the beginning, it is believed, to create a center of eclectic philosophy. For this purpose, he had the Athenian orator and statesman and his personal friend, Demetrius, invite the great minds, the philosophers of Greece, to teach or impart their knowledge to Alexandrian students. It was apparently the intention to classify such knowledge and select that which, in the opinion of Philadelphus and his associates, merited dissemination. The enthusiasm which the great school inspired in the seeking minds of the day altered the plans. The policy changed to research and advancement of knowledge, on the one hand, and on the other hand, a careful preservation in the great library of all the wisdom of all ages. Philadelphus became aware of the vast knowledge of natural law and of a Cosmic philosophy had by those who were initiates of the mystery schools. Much of such knowledge seemed to parallel that which he was having introduced in Alexandria from the West Ñ from Athens. His consequent actions prove that he realized that much of the Western knowledge was syncretic, and had formerly come from Egypt. In fact, Plato tells us that Solon got his information from the priests of Sais, who told him that all of the records weree preserved in the Temple of Neith. A further tradition relates that Solon, Thales, and Plato all visited the great college at Heliopolis, and that the last mentioned studied there.
A contemporary of Philadelphus was Manetho, a High Priest at Heliopolis, and a learned man, also a prominent scribe of the Great White Brotherhood, who had access to the secret teachings of the Order. Manetho was also master of the ancient Egyptian writing, or Hieroglyphics, which, in the Third Century B.C., was becoming archaic and could not be generally read. The Egyptians were at that time reading a modern version of the ancient writings, the Demotic, and Greek was becoming still more popular. Philadelphus commissioned Manetho to compile a history of Egypt, and particularly a text of the mystic philosophy of the Secret Schools of the Great White Brotherhood and Rosicrucians. This knowledge, we are told, was mainly contained in the Hieroglyphic inscriptions in the library of the priesthood at Ra. It will be recalled that Amenhotep IV (Akhnaton) declared Ra, the sun, to be a physical manifestation or symbol of the great sole God. This library, therefore, must have contained the great truths of his monotheistic religion, and the truths which the thinkers with which he surrounded himself at Tell el-Amarna discovered. Much that we know of the outer or profane history of Egypt came about through this compilation by Manetho. In fact, it is generally conceded that Plutarch acquired much of his information from this source. In a book of Manetho's, called Sothis, of which fragments only are to be found in the writings of others, appears the following letter to Philadelphus, from Manetho, telling of his efforts to compile the ancient wisdom: more.. Akhenaten
Born on the island of Sámos, Pythagoras was instructed in the teachings of the early Ionian philosophers Thales, Anaximander, and Anaximenes. At the age of eighteen he had absorbed all the learning which these great teachers of Greece could give him and it was about this time also that he had a vision in which he was shown in geometric lines the "key to the universe, the science of numbers, the rhythm and harmony of sacred numbers, the ternary law which rules the constellations and the septenary law which controls all evolution.
Stimulated by his vision he set off too Egypt to study under the Egyptian sages. At first the sages ignored him thinking that a Greek could not have the persistence to pursue deep study in the mysteries, but he persisted and they finally relented, and admitted him to their schools where he excelled. He passed all tests and initiations of the times.
Over a twenty-two year period he mastered all that the wise men had to teach him about sacred mathematics and the science of numbers. When he was about to return back to his home on Samos, the Persians overran Egypt and took Pythagoras and other Egyptian Magi prisoner to far-off Babylon.
Babylon in those days was a great metropolis in its heyday of great sages for in addition to their native Chaldean priests who were descendants of the Zoroasters, the Persians had captured Israelites from Palestine as well as the sages from Egypt. It was during this time that Pythagoras became exposed to all these other doctrines, religions, cults and magical practices. After absorbing all their teachings he knew more than anyone else about the eternal principles and laws and the science of numbers relating to the secrets of the universe.
From Grecian polytheism, Hindu trinitarianism, Persian dualism, and Hebrew monotheism he synthesized an esoteric science of numbers all his own, thus bringing into realization the vision he had had at eighteen.
After thirty-four years abroad, he obtained permission to return home to Samos where he intended to establish a School of Esotericism. But Samos proved too small and limiting so he and his mother moved to a city called Crotona in Italy around 530 BC where he established his school for initiates of the science of numbers. His admission standards were very rigorous, giving preference to the very young.
The school proved a success and his fame grew. He founded a movement with religious, political, and philosophical aims, known as Pythagoreanism. The philosophy of Pythagoras is known only through the work of his disciples. Pythagoras was not only an influential thinker, but also a complex personality whose doctrines addressed the spiritual as well as the scientific.
The Pythagoreans adhered to certain mysteries, similar in many respects to the Orphic mysteries. Obedience and silence, abstinence from food, simplicity in dress and possessions, and the habit of frequent self-examination were prescribed. He won many followers in the city of Croton itself and many from the nearby foreign territory, both kings and noblemen. What he said to his associates no-one can say with any certainty; for they preserved no ordinary silence.
Pythagoras, as we mentioned above, claimed that he had been Euphorbus, a warrior in the Trojan War, and that he had been permitted to bring into his earthly life the memory of all his previous existences. When he was staying at Argos he saw a shield from the spoils of Troy nailed up, and burst into tears. When the Argives asked him the reason for his emotion, he said that he himself had borne that shield at Troy when he was Euphorbus. They did not believe him and judged him to be mad, but he said he would provide a true sign that it was indeed the case: on the inside of the shield there had been inscribed in archaic lettering EUPHORBUS. Because of the extraordinary nature of his claim they all urged that the shield be taken down-and it turned out that on the inside the inscription was found. Consistent with his previous lives, the Pythagoreans taught the immortality and and transmigration of souls. Pythagoras seems to have been the first to introduce these doctrines into Greece.
Among the extensive mathematical investigations carried on by the Pythagoreans were their studies of odd and even numbers and of prime and square numbers. From this arithmetical standpoint they cultivated the concept of numbers, which became for them the ultimate principle of all proportion, order, and harmony in the universe. Through such studies they established a scientific foundation for mathematics. In geometry the great discovery of the school was the hypotenuse theorem, or Pythagorean theorem, which states that the square of the hypotenuse of a right triangle is equal to the sum of the squares of the other two sides.
The astronomy of the Pythagoreans marked an important advance in ancient scientific thought, for they were the first to consider the earth as a globe revolving with the other planets around a central fire. They explained the harmonious arrangement of things as that of bodies in a single, all-inclusive sphere of reality, moving according to a numerical scheme. Because the Pythagoreans thought that the heavenly bodies are separated from one another by intervals corresponding to the harmonic lengths of strings, they held that the movement of the spheres gives rise to a musical sound-the "harmony of the spheres."
As Pythagoras, Kuthumi laid the foundations of western
intellectual thinking. Can you imagine a world today without
numbers, without mathematics? Every object depends on some
measurement for its understanding. Kuthumi's work as Pythagoras
was to weave cosmic laws into the daily thinking of western
thinking. Slowly concepts such as the reincarnation of man's
soul, certain immutable mathematical principles, and the
origins of the earth through astronomy would form the basis
western thinking.
from.. "The Great Brotherhood of Light"
Pythagoras [Samos, 582 - 500 BC]
His immediate followers were strongly influenced by him, and even until today Pythagoras shines through the mist of ages as one of the brightest figures of early Greek antiquity. What he found out about triangles has been the beginning of mathematics in Western culture, and ever since mathematics -the art of demonstrative and deductive reasoning- has had a profound influence on Western philosophy, which can be observed down to Russel and Wittgenstein.
Pythagoras' influence found an expression in visual art and music as well, particularly in the renaissance and baroque epoch. The far-reaching imprint of his ideas is yet more impressive if we consider that he did not leave any original writings. Instead, all what is known about Pythagoras was handed down by generations of philosophers and historiographers, some of whom, like Heraclitus, opposed his views. In this light it is remarkable that Pythagoras' teachings have survived relatively undistorted until the present day.
Pythagoras was a native of the island of Samos. During his early life, Samos was governed by the powerful, unscrupulous tyrant Polycrates. Pythagoras did not sympathize with his government and thus emigrated to Croton in Southern Italy. Like the ancient Greek cities in Ionia, Croton was a flourishing commercial city that lived from importing and exporting goods. Obviously it was in Croton where Pythagoras developed most of his important ideas and theories.
Pythagoras founded a society of disciples which has been very influential for some time. Men and women in the society were treated equally -an unusual thing at the time- and all property was held in common. Members of the society practiced the master's teachings, a religion the tenets of which included the transmigration of souls and the sinfulness of eating beans. Pythagoras' followers had to obey strict religious orders where it was forbidden to eat beans, to touch white cocks, or to look into a mirror beside a light.
If all of this seems a bit odd, it might lead us to suspect that Pythagoras' personality reflects the inseparable blend of genius and madness that we associate with many other great men. It is said that once Pythagoras was walking up a lane in Croton when he came by a dog being ill-treated. Seeing this he raised his voice: "Stop, don't hit it! It is a soul of a friend. I knew it when I heard its voice." Spirits, ghosts, souls, and transmigration were obviously things he believed in deeply.
There was an opposition -if not rivalry- in ancient Greece between the gods of the Olymp and the lesser gods of more primitive religions. Pythagoras, like no other, embodied this contraposition of mystical and rational worlds, which is woven into his personality and philosophy. In his mind, numbers, spirits, souls, gods and the mystic connections between them formed one big picture. The following text tells the legend of his own existences:
"He was once born as Aethalides and was considered to be the son of Hermes. Hermes invited him to choose whatever he wanted, except immortality; so he asked that, alive and dead, he should remember what happened to him. Thus, in life he remembered everything, and when he died he retained the same memories. [...] He remembered everything - how he first had been Aethalides, then Euphorbus, then Hermotimus, then Pyrrhus, the Delian fisherman. When Pyrrhus died, he became Pythagoras." (Diogenes Laertius, Live of Philosophers, VIII 4-5)
"Pythagoras believed in metempsychosis and thought that eating meat was an abominable thing, saying that the souls of all animals enter different animals after death. He himself used to say that he remembered being, in Trojan times, Euphorbus, Panthus' son who was killed by Menelaus. They say that once when he was staying at Argos he saw a shield from the spoils of Troy nailed up, and burst into tears. When the Argives asked him the reason for his emotion, he said that he himself had borne that shield at Troy when he was Euphorbus.
They did not believe him and judged him to be mad, but he said he would provide a true sign that it was indeed the case: on the inside of the shield there had been inscribed in archaic lettering EUPHORBUS. Because of the extraordinary nature of his claim they all urged that the shield be taken down - and it turned out that on the inside the inscription was found." (Diogenes Laertius)
After Pythagoras introduced the idea of eternal recurrence into Greek thought, which was apparently motivated by his studies of earlier Egyptian scriptures, the idea soon became popular in Greece. It was Pythagoras' ambition to reveal in his philosophy the validity and structure of a higher order, the basis of the divine order, for which souls return in a constant cycle.
This is how Pythagoras came to mathematics. It could be said that Pythagoras saw the study of mathematics as a purifier of the soul, just like he considered music as purifying. Pythagoras and his disciples connected music with mathematics and found that intervals between notes can be expressed in numerical terms. They discovered that the length of strings of a musical instrument correspond to these intervals and that they can be expressed in numbers. The ratio of the length of two strings with which two tones of an octave step are produced is 2:1.
Music was not the only field that Pythagoras considered worthy of study, in fact he saw numbers in everything. He was convinced that the divine principles of the universe, though imperceptible to the senses, can be expressed in terms of relationships of numbers. He therefore reasoned that the secrets of the cosmos are revealed by pure thought, through deduction and analytic reflection on the perceptible world.
This eventually led to the famous saying that "all things are numbers." Pythagoras himself spoke of "square numbers" and "cubic numbers", and we still use these terms, but he also spoke of oblong, triangular, and spherical numbers. He associated numbers with form, relating arithmetic to geometry. His greatest discovery, the proposition about right-angled triangles, sprang from this line of thought:
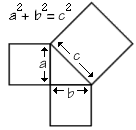 "The Egyptians had known that a triangle whose sides are 3,
4, 5 has a right angle, but apparently the Greeks were the first
to observe that 3²+4²=5², and, acting on this suggestion, to
discover a proof of the general proposition. Unfortunately for
Pythagoras this theorem led at once to the discovery of
incommensurables, which appeared to disprove his whole
philosophy. In a right-angled isosceles triangle, the square
on the hypotenuse is double of the square on either side.
"The Egyptians had known that a triangle whose sides are 3,
4, 5 has a right angle, but apparently the Greeks were the first
to observe that 3²+4²=5², and, acting on this suggestion, to
discover a proof of the general proposition. Unfortunately for
Pythagoras this theorem led at once to the discovery of
incommensurables, which appeared to disprove his whole
philosophy. In a right-angled isosceles triangle, the square
on the hypotenuse is double of the square on either side.
Let us suppose each side is an inch long; then how long is the hypotenuse? Let us suppose its length is m/n inches. Then m²/n²=2. If m and n have a common factor, divide it out, then either m or n must be odd. Now m²=2n², therefore m² is even, therefore m is even, therefore n is odd. Suppose m=2p. Then 4p²=2n², therefore n²=2p² and therefore n is even, contra hyp. Therefore no fraction m/n will measure the hypotenuse. The above proof is substantially that in Euclid, Book X." (Bertrand Russel, History of Western Philosophy)
This shows how Pythagoras' proposition immediately raised a new mathematical problem, namely that of incommensurables. At his time the concept of irrational numbers was not known and it is uncertain how Pythagoras dealt with the problem. We may suspect that he was not too concerned about it. His religion, in absence of theological explanations, had found a way to blend the "mystery of the divine" with commonsense rational thought.
From Pythagoras we observe that an answer to a problem in science may give raise to new questions. For each door we open, we find another closed door behind it. Eventually these doors will be also be opened and reveal answers in a new dimension of thought. A sprawling tree of progressively complex knowledge evolves in such manner. This Hegelian recursion, which is in fact a characteristic of scientific thought, may or may not have been obvious to Pythagoras. In either way he stands at the beginning of it.
"The Great Brotherhood of Light"
Kuthumi as Aethalides and Euphorbus
Scholars of ancient Greek philosophy mention that Pythagoras (see below) possessed a perfect recall of his former lives and introduced the idea of soul transmigration and reincarnation to the ancient Greeks. Pythagoras said he was once born as Aethalides who was considered to be the son of Greek God Hermes. Hermes invited him to choose whatever he wanted, except immortality; so he asked that, alive and dead, he should remember what happened to him. Thus in his life he remembered everything, and when he died he retained the same memories of previous lives.
Aethalides then died and reincarnated as Euphorbus, a warrior in the Trojan War. When Euphorbus died, his soul passed into one called Hermotimus. Hermotimus wanted to give a proof of his past lives and so went to Branchidae, entered the temple of Apollo and pointed to the shield which Menelaus had dedicated. He said that he had dedicated the shield to Apollo when he sailed back from Troy; it had by then decayed and all that was left was the ivory boss.
When Hermotimus died, he became Pyrrhus, the Delian fisherman; and again he remembered everything-how he had been first Aethalides, then Euphorbus, then Hermotimus, then Pyrrhus. When Pyrrhus died, he became Pythagoras and remembered everything also.
As so through Pythagoras and his verifiable past lives in Greek history the concept of reincarnation, one taken for granted in the East, made its inroads into the cradle of western philosophical thinking.
1. Who was Pythagoras?
2. What was the basis of his philosophy?
3. What are his and its connections with Freemasonry as we know it?
4. Can we from a study of these, formulate a code, and by following it, open up a path, whereby, if trodden by the individual student, he can, and should, reach that state, which, for want of a better name, we may call "Realisation", - the full knowledge of that which alone is real, - The Oneself?
I believe that all these questions can be answered and, tonight, I am going to make an attempt to condense the answers, as I see them, into one short paper. It would be impossible to go into each one fully, and, in process of condensation, the answers will overlap; but I will try to state them as simply as possible and I hope I may succeed in making the general outline, at least, clear. It can only be an outline, for that which must ultimately be sought is beyond form, formless. It can never be filled in fully in words. The connection with Freemasonry will, I think, make itself clear, if we attempt to answer the other three questions.
 First, then, just who was, or rather in,
Pythagoras. As the most famous of Greek
Philosophers, he was born at Samos about 586 B.C. His father was Mnesarchuss, a
man of learning and of noble birth. As a boy, Pythagoras had every advantage of
education and, later, seems to have travelled all over the world and to have formulated
his philosophy upon basic principles culled fron the various systems to which he gained
access. Thus he studied Astronomy and Astrology both in Chaldea and in Egypt, and
the Esoteric Sciences among the Brahmans of India. To this day his memory is
preserved in India under the name of Yavanacharya, the Ionian Teacher. Returning to
Europe, he settled at Crotona, in Magna Grecia, where he established a School, to
which were attracted all the best intellects of the civilized world. He left no writings
himself, so we have to piece together the details of his philosophy from the writings of
his followers.
First, then, just who was, or rather in,
Pythagoras. As the most famous of Greek
Philosophers, he was born at Samos about 586 B.C. His father was Mnesarchuss, a
man of learning and of noble birth. As a boy, Pythagoras had every advantage of
education and, later, seems to have travelled all over the world and to have formulated
his philosophy upon basic principles culled fron the various systems to which he gained
access. Thus he studied Astronomy and Astrology both in Chaldea and in Egypt, and
the Esoteric Sciences among the Brahmans of India. To this day his memory is
preserved in India under the name of Yavanacharya, the Ionian Teacher. Returning to
Europe, he settled at Crotona, in Magna Grecia, where he established a School, to
which were attracted all the best intellects of the civilized world. He left no writings
himself, so we have to piece together the details of his philosophy from the writings of
his followers.
To him we owe the word Philosopher. He was the first to teach the heliocentric system in Europe and no one of his time was so proficient in Geometry. Not only was he the greatest mathematician, geometer and astonomer of historical antiquity, but he also held highest place among scholars and metaphysicians. His fame cannot perish. He taught much of the Ancient Secret Wisdom, the truth of re-incarnation, the necessity for return to a natural system of diet, the rule of Justice in the whole Universe and the certainty of ultimate attainment of perfection by all beings. He realised that the solution of the great problem of Eternity belongs neither to religion, to superstition not to gross materialism. The harmony and balance of the two- fold evolution - of Spirit and of Matter, - have been made clear only in the Universal Numerals of of Pythagoras, who built his whole system entirely upon the so-called "Metrical Speech" of the Vedas.
In both Pythagorean and Brahman Philosophy the esoteric significance is derived from numbers. One of the few commentators who have paid just tribute to the high mental development of the old Greek and Latin writers, Thomas Taylor, says "Since Pythagoras, as Iamblichus informs us, was initiated in all the Mysteries of Byblus and Tyre, in the sacred operations of the Syrians and in the Mysteries of the Phoenecians, and also that he spent two and twenty years in the adyta of the Temples in Egypt, associated with the magicians of Babylon and was instructed by them in their venerable knowledge, it is not at all wonderful that he was skilled in Magic, or theurgy, and was therefore able to perform things which surpas merely human power, and which appear to be perfectly incredible to the vulgar."
For entrance to the School ot Pythagoras the qualifications were high and rigorously enforced and, once entered, the candidate came under very strict rules as regards diet, exercise and study. Besides this outer discipline there were pledged disciples who were expected to pass through three degrees, during a probation of five years. Of the outer disciples, leading an ordinary family social life, G.R.S. Mead says, "The authors of antiquity are agreed that this discipline had succeeded in producing the highest examples, not only of the purest chastity and sentiment, but also a simplicity of manners, a delicacy and a taste for serious pursuits which was unparalleled. This is admitted even by Christian writers". The three degrees of the Inner School were: HEARERS, who studied for three years in silence.
MATHEMATICI, learning Geometry and Music, the nature of Number, Form, Colour, Sound.
PHYSICI, who learned to master Cosmogony and Metaphysics. They were then prepared for the Mysteries.
The School at Crotona was closed at the and of the sixth century B.C., being persecuted by the Civil Power; but other communities carried on the tradition. Mead says that Plato intellectualized it to protect it from profanation, which was on the increase, and the Mysteries of Elusis, although they had lost its spirit and substance, still preserved some of its rites.
The root of all such teachings seems to have lain in Central Asia, whence Initiates spread to every land, carrying the same doctrines, using the same methods, working towards the same final goal. There was a common language and symbolism which served for intercommunication. Pythagoras in India received a high Initiation and later, Appolonius of Tyana followed in his steps. Typically Indian are the dying words of Plotinus, noblest of the Neo-platonists "Now I seek to lead back the self within me to the All-self." One great teacher has said, "The end of knowledge is to know God - not only believe; to become one with God - not only to worship afar off." We gain a hint in the Kathopanishat (V1- 17) "Let a man with firmness separate it (the soul) from his own body, as a grass stalk from its sheath," to which point we will return later.
Pythagoras gave the "knowledge of things that are" to his disciples and his knowledge of Music is said to have been such that he could use it to control men's wildest passions and to illuminate their minds. Iamblichus quotes instances and advises Porphyry to remove from his thoughts the image of the thing symbolised and to reach its intellectual meaning. Of the use of symbols Proclus remarks, "The Orphic method aimed at revealing divine things by means of symbols, a method common to all writers of divire lore." Great stress was laid upon the fact that numbers should be studied for the better comprehension of life, and not or use in commerce.
I am tempted to think that Pythagoras is a title, rather than a real name and it is significant that his father Mnesarchus, the nearest translation of which is "Ruler of Memory." Pythagoras, as a title, is identical, in root meaning, with Hiram Abif and with the Egyptian Thoth-Hermes. The root Pytha is the Sanscrit Pitta and the Latin Pater and the Greek, all meaning Father. It is again the same root as the Egyptian Phtha, one of the names of Thoth and Abif also means Father. Goras is the Sanscrit root Guru meaning Teacher, and the same root is found in Huram or Hiram. The Egyptian root is ChR Horus. ChR-Mes or Horus-Moses means Son of Horus. We may note here that Mercury, the latin equivalent of the Greek Hermes is a corruption of the Syrian Mar-Kurios meaning Son of the Lord.
The Pythagorean system of Cosmology is based upon the Decad, 10, or to use the name of the symbol associated with its name, the Tetractys. This Tetractys is represented in United Grand Lodge of England by a single great Hebrew Yod, or "I," placed immediately over the Grand Master's Throne, Yod being the tenth letter of the Hebrew alphabet and that also being its numerical value. The "pillar and circle," also 10, the perfect number of the Pythagoreans became later, among the Jews, a pre-eminently Phallic number, among whom it represented Jehovah as Male-Female. This Decad, representing the Universe and its evolution out of Silence and the Unknown depths of spirit, was presented to the student in Dual Aspect. It applied first to the Macrocosm, from which it descended to the Microcosm. To-day, upon four-square bases, we have, in our Lodges, or should have, two pillars, each bearing aloft a circle in perpetuation of this symbolism.
Both the purely intellectual and metaphysical, or "inner science" and the purely materialistic or "surface science", can be expounded by, and contained in, the Decad, study being by the deductive method of Plato or by the inductive method of Aristotle. Plato commenced with Divine Comprehension, and multiplicity proceded step by step from Unity, the digits appearing only to be returned to the Circle of the All-pervading Absolute. Aristotle started with perception by the senses, the Decad being regarded either as the unity which multiplies or as the matter which differentiates, its study being limited to two dimensions, to the Cross, or the 7; proceeding from the 10, the perfect number, on Earth as in Heaven. The whole conception apppears originally in India, but we cannot go into that now. The Western Teacher who first formulated it was Pythagoras.
Primarily numbers are symbols of the beginning and development of a universe, so the simplest way of bringing home to you their significance will be to take the first cycle of Creation, leading to full manifestation of the ultimate physical atom, and the building therefrom of matter, as we know it. I shall run through the stages very rapidly and leave it to you to go more fully into the subject should it appeal.
First, then, we have the Zero, nought, the Circle appears the Point at the Centre, potentiality, showing the Circle as not barren. In Arithmetic "0" is nothing, but, added to other numbers, is all things. Without it multiplicity cannot go beyond 9. This Circle-potential is the first number of the Cosmos, symbol of the Unknown, the Illimitable, containing all numbers as possibilities, as sunliqht contains all colours in whiteness.
The 0 the Circle or Ovum is Passive, and requires vivification before it can fructify and produce. The point, or centre, then becomes active and from it arises the Line, - the diameter which bisects the Circle, thereby polarising it. This is the Monad, the First Power of the Universe creating Polarity, opposites in Unity.
Some ancient philosophers spoke indiscriminately of Monad and One, but the Platonists drew sharp distinction, speaking of the Monad as that containing distinct yet profoundlly united multitude, whereas the One is the "sumnit of the Many" and simpler. One is the first of a series, nonexistant unless followed by other numbers, whereas the Monad includes all numbers, holds division in check. One is the apez of all numbers which spread fron it to the base, 10. Pythagoras realised the fundamental basis of numbers as Rhythm. In it was based the generation of all things. Numbers, to Pythagoras, were names and descriptions of Cosmic Ideas and Happenings.
One writer quotes him as saying, "There is a mysterious connection between the gods and numbers, on which the science of arithmancy is based. The soul is a world that is self moving; the soul contains in itself, and is, the quaternary, the tetractys, the perfect cube, and another says "Pythagoras is not reported as saying that the gods are numbers, or that all things are numbers, as some of his followers and critics affirm." Everything with the Pythagoreans, ideas, injustice, separation, mixture and even man and his horse, were all numbers" according to Aristotle. When speaking of the Monad or One, they actually referred to that which was before Creation, and, if philosophically minded, referred to it as the "Primordial Cross," if religiouis, as God, both understanding the same thing. They had many names for such number. Their One corresponds to the Advaitya, the one without a second of the Hindoos, creator and cause of all numbers.
The Duad, 2, is termed the cause of dissimilitude, matter. It is considered to be feminine, as the matrix or all things, and is the symbol of growth. Two cannot be produced from One, so duality is considered as the actual begining of manifestation; It is the drawing apart of God as Life and God as Substance, 1 X 1 is l and nothing but 1 so 1 needs 2, as Life needs Substance for manifestation and multiplication. 1 entering into relation with 2 gives rise to 3. Life, 1, ensouling Form, 2, becomes linked to it, 3, after being polarised, 2, from itself, 1. Opposites are essential to any creative purpose. 2 is therefore called the "First Number". Cornelius Agrippa calls it so because "it is the first magnitude and the common measure of all numbers, or, as the Pythagoreans term it, a confusion of unities. Thus, God, as One, the producer and clause of Persistence, polarizes, His Unity and draws apart from His substance, Subsistence, and, then vivifies it, producing Existence. 1 is potentially 2 for polarity is everywhere, as are pairs of opposites.
Avicebron of Cordova (1021-70) speaks of the affinity between "to be" and numbers and
says 3 is tte root of all things; for Spirit, 1, and Matter, 2, linked by Will, the bond
between, form the Triad. He ways, "All existing things are constituted after the nature
of numbers.....The Highest Abstract God is the indivisible, metaphysical unity". So 3, as
relating the action of the two opposites is rightly considered the number of true
beginning, without which no production is possible. One, potential, like a ring of
magnetized steel, is powerless until broken, or polarized, and the opposites are
themselves useless until there is a relation between them. 3 is then the number of active
growth and production. There are three distinct steps to be taken by the student before
he can enter the "outer court" of the Mysteries:-
1. He must collect together his forces and prepare to learn.
2. He must eliminate and subtract gross matter.
3. He must amalgamate or synthesise the result.
or in more familiar words
1. He must come of his own free will and accord.
2. He must be deprived of all metals and material valuables.
3. He must be properly prepared.
The third step of apprenticeship gains approbation from a master and leads the student
to a position where he can grasp the work with his whole nature.
The number 3 is most important and, masonically, so far as the Craft is concerned,
must be studied in conjunction with 5 and 7. I will return to this point.
The idea of the fundamental Trinity presupposes a condition of being before the worlds
were created.
4 is significant of system and order. Plutarch states that it is because of 4 that every body has its origin. It is Foundation, and does not relate to the building of physical forms and bodies, which is the function of 8, but to that of the Cosmic stones, the ultimate atoms out of which these forms will be built. Philo says it is the first number to show the nature of solidity. Mathematically it is Foundation, for, without it, no progression beyond 6 is possible, but with it completion in 10, that is, the complete cycle, can be reached. Three components blending equally gives 6 and no more but predomination of any one of them would lead to 7 or more, for 1 plus 2 plus 3 equals 6 and also 1 X 2 X 3 equals 6 each of which requires the addition of 4 to complete the cycle (or circle).
5 has a root meaning of "harvesting", the arranging in sheaves of produced substance, hitherto potential, now becoming matter. Five forms are combined in the foundation of the chemical atoms. It is a matter of rebirth and actual material commencement. That matter should be ensouled is not sufficient. Both matter and life must be qualified that gradation and diversity may result. Each chapter of the first ten chapters of Genises is said to refer to one of these numerical steps and it should be noted that chapter V contains a description of all emanated things and is devoted solely to generation.
D'Olivet reads it as a story of Cosmic generation. The Pythagorean name was cardiatis or cardialts, as the heart of things manifest, change of quality, the fire which "changes all things triply extended or which have length, breadth and depth into the sameness of a sphere and producing light." It is eminently a "circular number" and spherical, restoring itself in every multiplication. Note here the F.C's steps. By 5 arranging matter ready for use, three fundamental qualities are produced in the prepared matter and the three aspects of Diety find reflection in them, Will or Strength to Create, Love or Wisdom to Preserve, and activity or Beauty to Transmute or to send forth Creation, producing 6, representing that period in the creative process in which Triple spirit enters into Matter, already prepared as a triplicity to receive it. The double triangle is its symbol. Allendy defines it as a static correspondence between two analagous terms and not a transitory action or passage from one state to another. It is the instrument of progression but not the progression itself."
7 represents the progressive atomization of matter, without which building is impossible. The ancient Greeks called it Justice and represented it as a pair of scales, the bar pivoting about a point and supporting two hemispherical pans, each supported by 3 chains. 7 is to 3 as 3 is to 1. As 3 represents the development of a principle, so does 7 represent it doubly represented, that is to say not only manifested but objectively realised. Everywhere in nature we find this 7, in ourselves, in colour, music, the Arts, in healing and so on, balancing three on the life side against three on the form side with one giving synthosis.
Now, I think we may stop here, for this is the point to which the Craft of Freemasonry brings us. To complete the major cycle one has to consider the Holy Royal Arch and the Installation of W.M. which leads to it.
Before passing to one last point I want to take up, let me give the parallels briefly:- In the making of a Freemason there must first be the man himself, the Circle, No-number. Next comes that preparation in the heart which makes him the Circle-potential. The Unknown God, transcendent within-all men has become immanent in him. Then he takes his first step towards the door of the Lodge, The First step of a Series, he separates himself from the vulgar crowd and becomes a free unit, "Free and of Good Report." He becomes polarized, realising dimly that to is not only Body but also Spirit, he gains forward "In Strength." The E.A. degree is founded upon the number 3, and in it, by the union of his opposites, he makes production possible, he reaches "Plenty". In the F.C. degree he is able "To Establish" himself upon a sure foundation, begins to realise his real self. He gains control of matter and of "Worldly Possessions", producing multiplication of ports.
The M.M. degree is founded on the number Seven, which, so we saw represented full atomization. Here the One Rock of the Quarry has become the individualized multiplicity of prepared stones, ready for the building. Each is a complete work in itself but has to die as such in order to reach a reunion with the companions of its toil and take its place in the building of the Temple, the new cycle of 7 which it inaugurates.
Now for my last point. Several of the ancient Philosophers, including both both Plato and Aristotle, hint that man is something more than the three-dimensional being that he appears to be, at first sight. We cannot go into full evidence here, but Plato's beautiful allegory of the men chained in a cave with the light behind them and seeing only their own shadows and those of the passersby, thrown upon the flat surface of the opposite wall, should be called to mind. He tries to show how difficult it would be for one who had escaped and returned to his chained companions to bring to them any realisation of three dimensions. This seems to be a clear hint, and a study of Dimensional Masonry bears it out.
Before entering the Lodge for the first time, the Candidate is symbolically unaware of the existence of Spiritual Dimensions: Yes, in this three-dimensional world of ours, he has reached a stage where the unfolding of spiritual consciousness has become for him a definite aim. He has, in this sense, become one-pointed. So, when he comes to the door of the Lodge, he enters upon an undimensional Euclidean Point, having neither length, breadth nor thickness. Only at a later stage, when he has been restored to light, is it rivealed to him that this point was attached to and formed part of a straight line, a one-dimensional instrument, held by a brother whose grip was separated from it by a cross-piece, which, by its very position, indicated its two-dimensionality. Thus, the candidate transcended the first dimension of space and became a two-dimensional being.
Advancing to the E. A., he passes through a symbolic figure of 9, 12, and 15 units, indicating the Pythagorean proportion found in Euclid, 1, 47. Thus he surpases the second dimension of space and becomes a three-dimensional being capable of ruling and preparing a plate surface by knocking off all superfluous knobs and excrescences, roughly squaring the faces of the Ashlar in its rough form and preparing it for the hand of the more expert workman. This stone is placed upon the pedestal of the J.W. and should appear in the Ceremony in the N.E. corner of the Lodge.
Proceding onwards he enters upon the next stage upon an instrument which, although it is used upon three-dimensional work, is itself two-dimensional and which can be used to test the rectangularity of the previous advance. He then advances in a manner typical of three-dimensional motion. Under no conceivable circumstances can this advance take place in less than three dimensions. Now he produces a smooth stone, the Perfect Ashlar, which has place on the pedestal of the S.W. ard appears ceremonially in the S.E. corner of the Lodge.
Once more he passes on his way and enters upon, another stage of his quest, this time upon an instrument which is used in the depicting and measurement of the three- dimensional advance he has previously made. He now reaches the supreme test. Three stops he takes, each indicating an advance in a different direction and together showing that conquest of the three-dimensional world has been achieved. Then, boldly he marches forward, and indicates, in a very beautiful piece of symbolism, his passage into a new world, a world almost inconceivable to our untutored finite minds, the FOURTH DIMENSION of space. The Stone he can now prepare is of a shape normally outsite our consciousness.
It may be noted here that the W.T.'s in each degree of the Craft, and those of an I.M., indicate work in 1, 2, and 3 dimensions, the conquest, in each case, of the three boundaries of our three dimensional existence, length, breadth and thickness. This third stone is one over which there has been much wrangling, discussion and wild speculation, yet its essential qualities would seem to be sufficiently obvious. Most writers tacitly accept the Perfect Ashlar as the last possible stage in the preparation of of the stone, but this is true only of the three-dimensional world. If there are other dimensions, there will be further stages in the preparation, and it is significant that we find references to yet another stone, whose true place is on the Master's Pedestal, and, it its ceremonial position, "With the centre", perpendicular, perpendicular, or perpend, to three-dimensional space.
This is the PERPEND ASHLAR, and the reason why it cannot be seen in its completeness in the Lodge is that, existing in the Fourth Dimension, the only part we could perceive would be a perfect cube, suspended in space, to ever point to which it would be perpendicular. Mr. C.H. Hinton (in "The Fourth Dimension" calls it the Tessoract. It is to be noted that each Regular step is rectangular, taken symbolically at right angles to the last position. We move a point to produce a line; we move a line at right angles to the previous motion to obtain a superficies. This is the First Regular Step and from it we obtain a rectangular plane figure, a square; we now move our square at right angles to both the former directions of motion and the result is a solid cube, the Second Regular Step; and now we move this cube at right angles to all three directions of motion already used, and produce; by our Third Regular Step, a four-dimensionial figure, the Tesseract. Even then the journey is incomplete, for, as an I.M., the zealous brother uses tools belonging to the three dimensions of our space to prepare himself to work freely in the four-dimensional atmosphere of the Holy Royal Arch, wherein the whole scheme of Creation of Man as a reflection or form created by God "in the image of His own Eternity" and the method of the return of that image into the substance of T.G.A.O.T.U. in unfolded in the consciousness of the Initiate.
Thus far I have tried to answer the first three questions put at the beginning of this paper. Pythagoras, is, we have seen, fundamentally involved in our symbolism. We have taken a very hurried glance at the relevent portions of his Philosophy, and we have seen the same fundamentals running through our rituals. Now, very briefly to answer the fourth question.
We can, I think, say definitely that there is no Royal Road to Perfection. Each must find a way for himself. But signposts are not wanting, for to those who choose to raise their eyes from the plane of Matter, they point a clear way. The first and most important comes early in our Masonic knowledge." This can only come from the age-old three-fold method of advance being applied; CONCENTRATION, MEDITATION, CONTEMPLATION.
These we must apply daily to some portion of our Ceremonies, Tools and other Symbols, seeking ever to find their inner significance.
At no time in the Era of Recorded History has the application of these methods been more difficult than it is to-day in the Western world, but at no time has so much help been available to those who conscientiously attempt to apply them. In this Machine-tyrranized Age it is difficult to attain the necessary leisure, peace, quiet, stillness and silence, and the forgetfulness of the rush and hurry of the world in its search for the transient and worthless. Yet, even now, there are many signs that the world is getting tired of its own shallowness and sensationalism and is turning to things that are more worth while.
Perhaps the time is nearer than we think when men will at last seek the Middle Chamber of their own Temple, to find the wages of Truth. Tired of chasing an illusion, thay may seek the reality within, the Overself, which lies sheathed, as lies a grass-stalk in its husk, within the husk of Personality, ready to be drawn out into tho Light of T.G.A.O.T.U. Peace to All Beings, Amen.
The words of Josephus; But then for those that first
introduced philosophy, and the consideration of things
celestial and divine among them, such as Pherceydes the Syrian,
and Pythagoras, and Thales, all with one consent agree, that
they learned what they knew of the Egyptians and Chaldeans, and
wrote but little And these are the things which are supposed
to be the oldest of all among the Greeks; and they have much
ado to believe that the writings ascribed to those men are
genuine.
Flavius Josephus Against Apion:
Book 1 - Chapter 1
More clues to 'secret teachings'
..about Mount Carmel. Even by its very name, it is clear
that Mount Carmel has long been considered both a
natural paradise and a sacred mountain: for the literal Hebrew
meaning of "Carmel" is "garden paradise"
and the spiritual meaning of the word is defined in the
Metaphysical Bible Dictionary as,
"A place in consciousness where we realize the fullness of our
possibilities under the divine law. it is the garden
of God; Yahowshua called it 'paradise'. freedom from sense.
Mount Carmel stands for the center of spirituality,
which is located in man's body consciousness in the top of the
head."
In fact, Mount Carmel was considered so holy that there were strict taboos against spilling any blood there, not only of people but also animals, and it was even forbidden to harm a tree or deistub any of the natural life. These rules applied not only to the Essenes but also to anyone who climbed the holy mountain. Vulgar people were not permitted access to Mount Carmel.
Because the Essenes of Mount Carmel did not build permanent structures on the holy mountain that no - or very little physical trace of their presence remains for archaeologists to study.
In The Life of Pythagoras written in the 2nd century by Jamblichus, we learn that the great sage Pythagoras, as a young seeker, visited the Essene sanctuary atop Mount Carmel.
"In Phoenicia he [Pythagoras] conversed with the prophets who were descendants of Moses. After gaining all he could from the Phoenician mysteries, he found that they had originated from the sacred rites of Egypt. This led him to Egypt. Following the advice of his teacher Thales, he left through the agency of some Egyptian sailors, and landed on the coast under Mount Carmel."
Pythagoras then climbed Mount Carmel where he evidently received powerful teachings from the Essene Nazarenes; for when he returned to the ship, the sailors, who had hatched a secret plan to sell young Pythagoras into slavery, were mesmerized by his spiritual luminosity and unable to harm him. Whatever teachings Pythagoras had studied and practiced during his stay with the Essenes atop Carmel, they had obviously served to transform him!
He climbed the mountain as an advanced seeker, he decended the mountain A MASTER.The sailors, reports Jamblichus, believed Pythagoras had become "supernatural" We know that Pythagoras went on to become a chief exponent of vegetarianism, reincarnation, fasting and sacred geometry - all Essene teachings - and that he and his followers wore only white linen garments, in the fashion of the Essenes of Mount Carmel.
Josephus, writing between A.D. 75 and A.D.85, tells us
that the Essenes were Pythagorean in lifestyle.
"The sect of the Essenes maintain that Fate governs all things,
and that nothing can befall man contrary to its determination
and will. These men live the same kind of life which among the
Greeks has been ordered by Pythagoras."
500BC. The Pythagoreans believed in the Eastern idea that the soul is divine and immortal, and that it does reincarnate after each death. It is imprisoned in our imperfect material bodies as a punishment. The goal for all rational people is to break free from this prison.
Pythagoras was a philosopher. In addition to his beliefs
about numbers, geometry and astronomy described above, he held
the following philosophical and ethical teachings:
Without narrating a life of Pythagoras, there are two
important things to remember about him. First Pythagoras was an
initiate of all the ancient mysteries. This fact is reflected
first hand in The Life of
Pythagoras by Iamblichus (B.250 AD).
After receiving all the available teachings of ancient Greece
and presumably initiated into the Eleusinian mysteries and after
consulting all the Greek oracles, he travelled to Egypt on the
advice of his teacher, Thales. Here, he was initiated into the
mysteries of Isis at the hands of priests at Memphis and Thebes.
Like his future successor Plato, he was placed on the Tau wooden cross and raised on the third day from the granite sarcophagus in the Kings chamber. Later, he travelled to Phoenicia and Syria where he was initiated into all the mysteries of Adonis at Byblos and Tyre. Taken captive by the soldiers of Cambyses, he was carried off to Babylon, where he was later initiated by the Magi in the Chaldean lore of astronomy and geometry. Although Iamblichus does not mention this sojourn, he is said to have travelled through Media and Persia into India where he studied for several years as a pupil of the Brahmin priests of Elephanta and Ellora. The name of Pythagoras is still preserved in Brahmin records as Yavancharya, the Ionian teacher.
Second, as a great initiate, Pythagoras was part of the messianic cycle leading to the birth of Christ. R. Steiner has spoken on numerous occasions that the messianic cycle preparing for the birth of Christ was more than the scripture of the Jewish-Hebrew tradition dating from Moses and the Exodus. In fact, all the ancient mystery schools contributed through their mystery wisdom and rites of initiation to the birth, life and death of Christ at Golgatha. A glimpse of this secret knowledge was discussed supra when it was related how the Egyptian initiation in the King's chamber enacted the drama of Christ as nailed to the Tau cross, death in the crypt for three days and resurrection to a new spiritual life.
Another glimpse of this secret key known to ancient mystery
wisdom has been revealed by Steiner in a series of lectures on
the birth of the Christ child as follows....
About 100 years
prior to the birth of the Christ child, an incarnation of the
Gautama Buddha was born in the Holy Land named Jesus Ben (son of)
Pandira. He became the founder of the Essence religions sect,
which later taught the Christ child after he left the temple at
age twelve. His purpose was to prepare the world for the birth
of Christ. He had a pupil named Matthew whose name passed over
to other pupils and the Matthew Synoptic gospel was written in
the form of rituals taken from the ancient mysteries by the
first Matthew. (This is why the Matthew gospel was first
written in Hebrew and not Greek as translated by St. Jerome.)
The good Madame H.P.B. confirms this historical event in her paper entitled, The Esoteric Character of the Gospels, referring to the Rabbinical tradition in the Babylonian Gemera called Sepher Toldus Jeshu about Jesus being the son of one named Pandira, and having lived a century earlier than the Christian era, namely, during the reign of the Jewish King Alexander Jannaeus and his wife Salome who reigned from 106 to 79 B.C. "accused by the Jews of having stolen from the Holy of Holies the Incommunicable name, Jehoshua (Greek for Jesus) was put to death by the Sanhedrin at Lud. He was stoned and then crucified on a tree, on the eve of Passover. The narrative is ascribed to the Talmudistic authors."
This story relates; In the Bible, Jesus descended from the House of David. However, two Jesus children were born, one from the Solomon line of the House of David and one from the Nathan line of the House of David. The individuality who was the Solomon Jesus child was an incarnation of Zarathustra; the founder of the cultural epoch of Persia and the mystery school of the Magi. At age twelve, through an occult (secret) process, the individuality of Zarathustra forsook the body of the Solomon Jesus child and went over to the etheric body of the Nathan Jesus child. The body of the Nathan Jesus child was prepared by and contained the astral body of the Gautama Buddha. After leaving the temple at age twelve, the now Jesus of Nazareth child, bearing the etheric body of Zarathustra and the astral body of Buddha, wandered the near east visiting the sites of the ancient mysteries, including the Mithras cult. I say the now Jesus of Nazareth because after age twelve, the mother of the Nathan Jesus boy died and the father of the Solomon Jesus boy died. The surviving spouses married and the brothers and sisters of the Solomon child became part of his family, now living in Nazareth.
Later, Jesus of Nazareth spent many years with the Essene sect, where he met John the Baptist. At the baptism of Jesus in the Jordan, the ego of Christ as solar logos descended upon him completing the four bodies of his lower quaternary.
Knowing the Zarathustra connection in the Messianic cycle, the Pythagoran connection can be explained. Porphyry (b.233 A.D.) the Greek neoplatonic philosopher in writing an the life of Pythagoras says that, "In Babylon he associated himself with the other Chaldeans, especially attaching himself to Zaratus (Zarathustra), by whom he was purified from the pollution of his past life...and here, Pythagoras acquired the greater part of his wisdom." The name Zarathustra as well as the names of other ancient savants like Mani, Manu, Hermes, Rishi, etc. once stood for an original person, but in the mystery tradition, the students take the name of the teacher, so that after several generations, the name becomes generic representing an esoteric stream of knowledge. This is why modern scholars can never exactly date the life and death of the great adepts.
Steiner says that Zarathustra incarnated as Zaratus (as per Porphyry) or Nazarathes, the teacher of Pythagoras in Babylon. Here, in Babylon, Pythagoras became initiated in the Messianic cycle as did the Jews of the Babylonian captivity. In subsequent incarnations, Pythagoras was reborn as one of the three Magi who visited the crib of Jesus in Bethlehem and later became a disciple of Jesus of Nazareth.
The good Madame H.P.B. also confirms the Zarathustra connection in the Messianic cycle. The first three chapters of Vol II, ISIS UNVEILED is dedicated to the proposition that the appellation of Jesus of Nazareth was so called not because he came from his father's residence at Nazareth but because Jesus was an initiated member of the esoteric mystery sect called the NAZARIA, the healers.
To support this theory, she states that the Nazireate sect existed long before the Laws of Moses and originated among peoples most inimical to the "chosen" ones of Israel, the people of Galilee, where was built a city called Nazara, the present Masra. Here, and in the caves of Bethlehem, the Nazra held their sacred mystery rites of initiation. In fact, history shows the first Christian sects to be either the Ebionites or the Essenes, the later being Therapeutae healers, of which the Nazarenes were a dissenting branch which included John the Baptist and his followers.
The Madame says that the Nazara of Galilee were first Pythagoreans and after conversion to the Buddhist monastic life, became the sect known as Essenes. The word "Nazar" means to vow or consecrate oneself to the service of God. As a noun it was an emblem of such dedication, and for this reason Joseph, Ezra, Sampson and Samuel were called Nazars. She concludes that the Zar-adas of the Pythagorean biographer Porphyry was the head or Nazar of the Babylonian mystery school in which Pythagoras was initiated. She, like Steiner, recognizes that the word Zoroaster becomes a generic term since the followers of the original Zoroaster called themselves as disciples by the same name.
Thus, some historians refer to Pythagoras Babylonian teacher as Nazarathus (by Clement of Alexandria) or Zaratus or Zaradas or Narazad. These are but changes of idioms for different cultures. The key is that they are all derivatives of the word Nazar which establishes a direct link with Pythagoras, his teacher Zarathustra at Babylon and the Essene sect which instructed Jesus prior to his leaving this sect with John the Baptist.
In addition, other clues emerge with the Nazarene connection with Christ. For example, the Nazarene priests and initiates were circumcised. All sect members were baptized in the Jordan River and before and after baptism, they had to fast. The gospels tell us that Jesus fasted for forty days after his baptism in the Jordan by John. The Nazarenes wore their hair long, like Sampson and Elijah, the prophet. Both John the Baptist and Jesus are described in the gospels as wearing very long hair. Lastly, Jesus is described as wearing a log white garment without any seams. This is the same description given by Josephus of Nazarene priests and the Buddhist Essenes. More...from Pythagoras
In conclusion, Pythagoras, the great initiate, carried forth his occult wisdom, hand in hand with Zarathustra, in the ancient mysteries preparation of the Messianic cycle.
Like Thales, Pythagoras is rather known for mathematics than for philosophy. Anyone who can recall math classes will remember the first lessons of geometry that usually start with Pythagoras famous proposition about right-angled triangles: a²+b²=c². Pythagoras found this principle two and a half millennia ago -around 532 BC- and with it his name and philosophy have survived the turbulences of history.
Amazing Facts! Hebrew Number codes as they apply to Masonry
In Masonic ritual the Lost Master's Word is frequently explained to refer to 'The marrow of the bone'. This is interpreted to mean life-force or virile vigour - which is especially apt, as its value of 1520 equates it with both the fertile procreative energy of 'The Vernal Equinox' and the fertilising instrument O IquV FalloV.
It is possible that AVN was the original Master's Word. It has the significant value of 707: this represents half of the square root of two. The ancient importance of this number is testified to by our finding O QeoV ErmhV - The God Hermes, and ParqenoV Mariam - The Virgin Mary, equally valued.
It is also a fact that 153 is the value of H Magdalhnh - The Magdalene. Mary Magdalene is very closely associated with the resurrection of Jesus, as she was the first to discover the empty tomb and encounter his risen form. If the resurrection is viewed in terms of the Greek mysteries, she would have been acting in the role of initiatrix - like Isis raising her dead husband Osiris.
756 is also the value of the village EmmaouV, which was where Jesus first appeared to his disciples after his resurrection (Luke 24, 13). Furthermore, the Greek spelling of Hiram - Ceiram - has this value, and the word AparabatoV meaning 'imperishable'. One could add that a vesica 756 in width has a circumference of 3168 (the perimeter of the archetypal temple and value of KurioV IhsouV CristoV - Lord Jesus Christ) and that 756 is the side length of the Great Pyramid as measured in feet.
It is also worth pointing out that the Hebrew word for 'marrow' MCh is an
anagram of ChM - Ham, the son of Noah. Ham represents the sun in Masonic ritual at
the midheaven station. Notice too that 'Ham' is 'Mah' in reverse. The names of father and
son are written NCh and ChM and have a combined value of 58 + 608 = 666. This is the
supreme solar number. Their lower value of 58 + 48 = 106 is equal to the lower value of MVABAVN.
So Much for the christian 666 Satan theory!
Return to.. Passage 3
| Solomon's Goddess Worship | Solomons Temple - The Builders | The connection to the Great Mystery Religion of the ancients, The Great Pyramid of Egypt | or visit the Island of
Atlantis,
The Connection |
Site Map | The Real Jesus | Gnostic Truth carried on by Jesus | Back to The Widow's Son | The Lies of the so-called prophet Paul | The Murder of the disciples | The False Church | The Magi |
NO PART OF THIS SITE MAY BE COPIED OR REPRODUCED, IN WHOLE OR IN PART WITHOUT THE EXPRESS WRITTEN APPROVAL OF THE WEBMASTER... © COPYRIGHT 1999 - 2008 C.I.C.ALL RIGHTS RESERVED